Sample automatic measurement method (2)

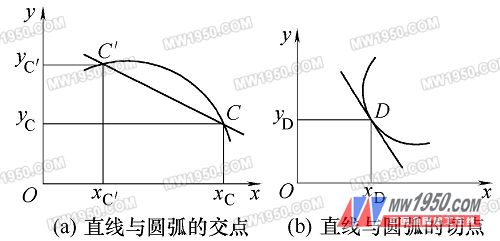
Figure 3 The intersection of a line and an arc

The equations for lines and arcs are established by the known two points on the line being measured and the known three points on the arc. The measurement method and program operation are to collect N points (N ≥ 3) on the straight line and the arc of the sample.

If the line intersects the arc, the coordinates of the intersection are displayed and automatically numbered and the distance between the intersection and the center of the circle is given; if the line is tangent to the arc, the coordinates of the tangent are given and automatically numbered and given the center to the line Distance; if the line is separated from the arc, the distance from the center of the circle to the line is given.

(4) The intersection of the arc and the arc (cut) and the center of the arc (see Figure 4) are similar to the straight line and the arc. There are also three cases of arc and arc, that is, the arc is separated from the arc. Tangent, intersecting.

The equation of the arc is established by the known three points on the measured arc. The measurement method and program operation are to collect N points (N ≥ 3) on the measured arc of the template.

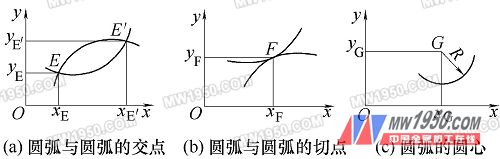
Figure 4 The intersection of the arc and the arc (cut) and the center of the arc

(5) The intersection and tangent point of the straight line, arc and arbitrary function curve.

(6) The intersection and tangent point of the two arbitrary function curves.

The above two items (5) and (6) will be discussed in the measurement examples.

It should be pointed out here that in order to improve the measurement accuracy, when collecting straight lines and arcs, some points can be taken, without being limited by two-point lines and three-point circles. The software uses the least squares method for both lines and circles. .

Finally, it should be pointed out that when the sample is measured, the measurement plan should be determined according to the geometric elements of the profile. What points should be taken, which points are used to establish the line? Which points are used to establish the circle?... Then, the coordinates are then calculated. Point acquisition, finally call the required software function, input the parameters according to the program, and get the final measurement result.

2. Determination of the intersection size

The intersection size refers to the distance from the intersection point to the reference edge of the template or the distance between the intersection point to a specified point (such as the center of the arc), that is, the size of the sample point to point, point to line.

It is very easy to measure the sample intersection size on a microcomputer-based display because it can be processed automatically by software. Software processing functions include: coordinate transformation, two-point and midpoint coordinates, point line spacing, straight line angle, N-point circle, circle and circle, and so on.

3. Measurement examples

As shown in Figure 5a, the measurement and solution are required: the coordinates of the intersection of the two lines, the angle of the intersection and the size of the intersection; Figure 6a requires measurement and solution: the coordinates and size of the intersection of the line and the arc; Figure 7a requires measurement and solution: arc The coordinates of the intersection with the arc, the radius of the arc, and the coordinates of the center of the circle.

Previous page next page